Biggest Paradoxes of the Modern World

Paradoxes that will shock you into Confusion

A Paradox may be a statement or drawback that either seems to supply 2 entirely contradictory (yet possible) outcomes, or provides proof for one thing that goes against what we have a tendency to intuitively expect. Paradoxes are a central a part of philosophical thinking for hundreds of years, and square measure continuously able to challenge our interpretation of otherwise straightforward things, turning what we'd suppose to be true on its head and presenting America with incontrovertibly plausible things that square measure if truth be told even as incontrovertibly not possible. Confused? you ought to be.

THE BOOTSTRAP contradiction in terms

The Bootstrap contradiction in terms could be a contradiction in terms of your time travel that queries however one thing that's taken from the long run and placed within the past may ever acquire being within the initial place. It’s a typical figure utilized by phantasy writers and has galvanized plotlines in everything from Doctor United Nations agency to the Bill and Ted movies, however one in every of the foremost unforgettable and simple examples—by academician David Toomey of the University of Massachusetts associate degreed employed in his book The New Time Travellers—involves an author and his manuscript.

Imagine that a time person buys a replica of Hamlet from a shop, travels back in time to Elizabethan London, and hands the book to William Shakespeare, United Nations agency then copies it out and claims it as his own work. Over the centuries that follow, Hamlet is reprinted and reproduced innumerous times till finally a replica of it finishes up back within the same original shop, wherever the time person finds it, buys it, and takes it back to William Shakespeare. Who, then, wrote Hamlet?

The crocodile reptile Paradox

A crocodilian reptile snatches a young boy from a riverside. His mother pleads with the crocodilian reptile to come back him, to that the crocodilian reptile replies that he can solely come back the boy safely if the mother will guess properly whether or not or not he can so come back the boy. there's no downside if the mother guesses that the crocodilian reptile can come back him—if she is true, he's returned; if she is wrong, the crocodilian reptile keeps him. If she answers that the crocodilian reptile won't come back him, however, we tend to find yourself with a paradox: if she is true and also the crocodilian reptile ne'er meant to come back her kid, then the crocodilian reptile must come back him, however in doing thus breaks his word and contradicts the mother’s answer. On the opposite hand, if she is wrong and also the crocodilian reptile really did will come back the boy, the crocodilian reptile should then keep him even supposing he meant to not, thereby conjointly breaking his word.

The crocodilian reptile contradiction is such associate degree ancient and enduring logic downside that within the Middle Ages the word "crocodilite" came to be accustomed talk over with any equally brain-twisting quandary wherever you admit one thing that's later used against you, whereas "crocodility" is associate degree equally ancient word for faultfinding or fallacious reasoning

THE POTATO Paradox

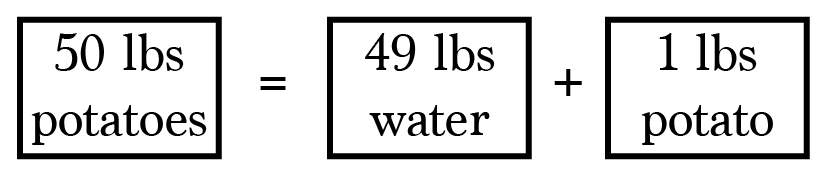
Imagine that a farmer features a sack containing one hundred lbs of potatoes. The potatoes, he discovers, square measure comprised of ninety nine water and 1 Chronicles solids, thus he leaves them within the heat of the sun for daily to let the quantity of water in them cut back to ninety eight. however once he returns to them the day once, he finds his one hundred avoirdupois unit sack currently weighs simply fifty lbs. however will this be true? Well, if ninety nine of one hundred lbs of potatoes is water then the water should weigh ninety nine lbs. one/2|the half of|the half} of solids should ultimately weigh simply 1 avoirdupois unit, giving a magnitude relation of solids to liquids of 1:99. however if the potatoes square measure allowed to dehydrate to ninety eight water, the solids should currently account for two of the weight—a magnitude relation of 2:98, or 1:49—even although the solids should still solely weigh 1lb. The water, ultimately, should currently weigh 49lb, giving a complete weight of 50lbs despite simply a tenth reduction in water content. Or should it?

Although not a real contradiction in terms within the strictest sense, the unreasonable Potato contradiction in terms may be a notable example of what's referred to as a real contradiction in terms, within which a basic theory is taken to a logical however apparently absurd conclusion.

THE DICHOTOMY PARADOX

Imagine that you’re about to set off walking down a street. To reach the other end, you’d first have to walk half way there. And to walk half way there, you’d first have to walk a quarter of the way there. And to walk a quarter of the way there, you’d first have to walk an eighth of the way there. And before that a sixteenth of the way there, and then a thirty-second of the way there, a sixty-fourth of the way there, and so on.

Ultimately, in order to perform even the simplest of tasks like walking down a street, you’d have to perform an infinite number of smaller tasks—something that, by definition, is utterly impossible. Not only that, but no matter how small the first part of the journey is said to be, it can always be halved to create another task; the only way in which it cannot be halved would be to consider the first part of the journey to be of absolutely no distance whatsoever, and in order to complete the task of moving no distance whatsoever, you can’t even start your journey in the first place.